___________________________________________________________________
Calculus  II                                              Test #3                                   April 19, 2004

Name____________________         R.  Hammack                               Score ______
___________________________________________________________________

(1) Find the following integrals.

(a) ∫  1/(4 - x^2)^(1/2) dx =sin^(-1)(x/2) + C


(b) ∫  tan^2(x) dx = tan(x) - x + C


(c) ∫  x sin(5x) dx = x(-1/5cos(5x)) - ∫ ( -1/5cos(5x)) dx = -(x cos(5x))/5 + sin(5x)/25 + C

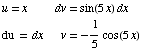
u = x              dv = sin(5x) dx  du = dx         v = -1/5cos(5x)



(d) ∫  sin(ln(x))   dx =x sin(ln(x)) - ∫x cos (ln (x)) 1/x dx = x sin(ln(x)) - ∫ cos (ln (x)) dx

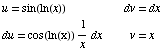
u = sin(ln(x))              & ... ) 1/x dx              v = x

Now we use integration by parts a second time on the most recent integral.

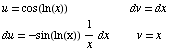
u = cos(ln(x))              & ... ) 1/x dx              v = x

∫ cos (ln (x)) dx = cos(ln(x)) x - ∫x(-sin (ln (x)) 1/x) dx = cos(ln(x)) x + ∫sin (ln (x)) dx

Plugging THIS back into the first line gives

∫  sin(ln(x))   dx = x sin(ln(x)) - (cos(ln(x)) x + ∫sin (ln (x)) dx)

From this we get
2∫sin(ln(x))    dx = x sin(ln(x)) - cos(ln(x)) x

so ∫sin(ln(x)) dx = 1/2x ( sin(ln(x)) - cos(ln(x))) + C



(e)  ∫_0^( π/2) cos^3(x) dx =∫_0^( π/2) cos^2(x) cos(x) dx = ∫_0^( π/2) (1 - sin^2(x)) cos(x) dx = ∫_sin(0)^( sin(π/2)) (1 - u^2) du =[u - u^3/3] _0^1 = 2/3


(f)  ∫  tan^2(x) sec^2(x)   dx =∫   (tan(x))^2sec^2(x)   dx = ∫  u^2  du = u^3/3 + C = tan^3(x)/3 + C



(g)  ∫    (9 - x^2)^(1/2) dx =   ∫    (9 - (3sin(θ))^2)^(1/2) 3cos(θ) dθ = ∫ &nb ... 3cos(θ) dθ = 9∫ cos^2(θ) dθ = 9/2 (θ + cos(θ) sin(θ)) + C


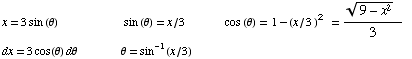
x = 3sin (θ)             &nbs ... sp;            θ = sin^(-1)(x/3)


Converting the above expression back to x, we get:

9/2 (sin^(-1)(x/3) + (9 - x^2)^(1/2)   /3x/3) + C = 9/2sin^(-1)(x/3) + (x (9 - x^2)^(1/2)   )/2 + C



(h)  ∫ (x^2 - 1 )^(1/2)/x   dx = ∫ (sec^2(θ) - 1 )^(1/2)/sec(θ) sec(θ) tan(θ) dθ = ∫tan^2( ... #8747; (sec^2(θ) - 1) dθ = tan(θ) - θ + C = (x^2 - 1 )^(1/2) - sec^(-1)(x) + C


x = sec(θ)               ... ;           tan(θ) = (x^2 - 1 )^(1/2)


(i) ∫ (2x + 10)/(x^2 + 10x + 24)   dx =∫ (2x + 10)/((x + 4) (x + 6))   dx = ∫ 1/(x + 4) + 1/(x + 6)   dx = ln | x + 4 | +ln | x + 6 | +C


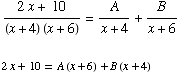
(2x + 10)/((x + 4) (x + 6)) = A/(x + 4) + B/(x + 6)   2x + 10 = A (x + 6) + B (x + 4)

Set x = -6,  get  2(-6)+10 = -2B   ====>  B=1
Set x = -4,  get  2(-4)+10 = 2A   ====>   A=1



(j) ∫   (x^2 + 2)/(x + 2)   dx = ∫ x - 2 + 6/(x + 2)   dx = x^2/2 - 2x + 6ln | x + 2 | +C


Doing the long division:

               &nbs ... ;                6

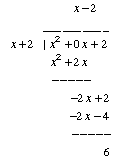
Therefore (x^2 + 2)/(x + 2) = x - 2 + 6/(x + 2)


(2) Evaluate the following improper integrals.

(a)   ∫_2^∞2/x^2 dx =Underscript[lim , l∞] ∫_2^l2/x^2 dx = Underscript[lim , l∞][-2/x] _2^l = Underscript[lim , l∞] (-2/l - -2/2) = 0 + 1 = 1


(b)   ∫_0^1x^(-1/3) dx =Underscript[lim , l0^+] ∫_l^1x^(-1/3) dx = Underscript[lim , l0^+][(x^(1/3))^2/(2/3)] _l^1 = Underscript[lim , l0^+] ((1^(1/3))^2/(2/3) - (l^(1/3))^2/(2/3)) = 3/2


(3) Evaluate the limits.

(a) Underscript[lim , x⟶0^+] ln(x)/(x - 1) =∞ NOTE: This is NOT an indeterminate form, so L'Hopital's Rule does not apply


(b)   Underscript[lim , x⟶∞] (1 - 3/x)^x = Underscript[lim , x⟶∞] e^ln((1 - 3/x)^x)    = Underscript[lim , xϿ ... ^2/(1 - 3/x)/(-1/x^2)) = e^(  Underscript[lim , x⟶∞] -3/(1 - 3/x)) = e^(-3)